Difference between revisions of "$$\eta$$ scaling for dynamical nhyp"
| (8 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | We performed a scaling test of the $\eta$ parameter on a single $24^3 \ | + | We performed a scaling test of the $$\eta$$ parameter on a single $$24^3 \times48$$ nhyp dynamical lattice. We want to determine a regime of $\eta$ values where the $$\eta^2$$ scaling of the mass shift is valid. As we go to smaller values of $\eta$ numerical limitations will begin to arise. We want to find where this break down occurs. We did the analysis for 2 different precisions for the CG inverter: $$10^{-8}$$ and $$10^{-13}$$. |
| − | For the $10^{-8}$ precision we ran the simulation for 10 values of $\eta$ starting from $\eta_0 = 3.0\times 10^{-5}$. The subsequent values of $\eta$ are in powers of 2, i.e. $\eta_1 = 2^1 \eta_0$, $\eta_2 = 2^2 \eta_0$ etc. For the $10^{-13}$ we ran the simulation for 16 values of $\eta$ starting from $\eta_0 = 4.6875\times 10^{-7}$. This value is chosen such that $2^7( 4.6875\times 10^{-7}) = 3.0\times 10^{-5}$. | + | For the $$10^{-8}$$ precision we ran the simulation for 10 values of $$\eta$$ starting from $$\eta_0 = 3.0\times 10^{-5}$$. The subsequent values of $$\eta$$ are in powers of 2, i.e. $$\eta_1 = 2^1 \eta_0$$, $$\eta_2 = 2^2 \eta_0$$ etc. For the $$10^{-13}$$ precision we ran the simulation for 16 values of $$\eta$$ starting from $$\eta_0 = 4.6875\times 10^{-7}$$. This value is chosen such that $$2^7( 4.6875\times 10^{-7}) = 3.0\times 10^{-5}$$. |
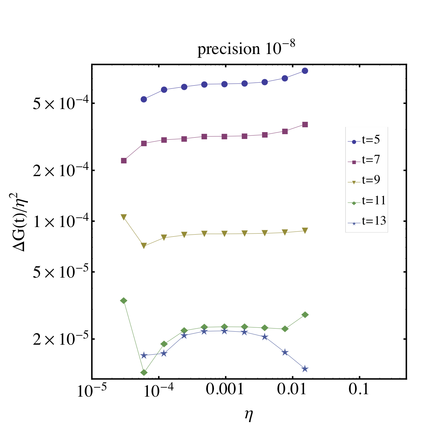
| − | We plot $(G_E(t) -G_0(t))/\eta^2$ as a function of $\eta$. We expect a constant behavior in the region where the $\eta^2$ scaling is valid. The | + | We plot $$(G_E(t) -G_0(t))/\eta^2$$ as a function of $$\eta$$. We expect a constant behavior in the region where the $$\eta^2$$ scaling is valid. The plots below show our results for the neutron at several different time slices. The parameters used in this analysis are listed in the table below. |
| − | ==='''Configuration | + | ==='''Configuration'''=== |
| − | + | knhyp242448_beta7.1_kappa0.1282_023 | |
| − | + | ||
| + | ran on Fermilab. | ||
{| class="wikitable" style="text-align: center;" | {| class="wikitable" style="text-align: center;" | ||
| Line 15: | Line 16: | ||
! CG precision !! 1e-8 !! 1e-13 | ! CG precision !! 1e-8 !! 1e-13 | ||
|- | |- | ||
| − | ! $\kappa$ | + | ! $$\kappa$$ |
| 0.1282 || 0.1282 | | 0.1282 || 0.1282 | ||
|- | |- | ||
| − | ! n iterations | + | ! n iterations ~ |
| − | | | + | | 700 ||1250 |
|- | |- | ||
! timing for 1 inversion ~ | ! timing for 1 inversion ~ | ||
| − | | | + | | 15 sec || 22 sec |
| + | |- | ||
| + | ! N GPUs | ||
| + | | 2 || 4 | ||
|} | |} | ||
| + | |||
| + | ==='''Plots'''=== | ||
| + | [[File:Etas_1e-8.png|left|430px]] | ||
| + | [[File:Etas_4.6875e-7.png|right|430px]] | ||
Latest revision as of 22:59, 2 April 2020
We performed a scaling test of the $$\eta$$ parameter on a single $$24^3 \times48$$ nhyp dynamical lattice. We want to determine a regime of $\eta$ values where the $$\eta^2$$ scaling of the mass shift is valid. As we go to smaller values of $\eta$ numerical limitations will begin to arise. We want to find where this break down occurs. We did the analysis for 2 different precisions for the CG inverter: $$10^{-8}$$ and $$10^{-13}$$.
For the $$10^{-8}$$ precision we ran the simulation for 10 values of $$\eta$$ starting from $$\eta_0 = 3.0\times 10^{-5}$$. The subsequent values of $$\eta$$ are in powers of 2, i.e. $$\eta_1 = 2^1 \eta_0$$, $$\eta_2 = 2^2 \eta_0$$ etc. For the $$10^{-13}$$ precision we ran the simulation for 16 values of $$\eta$$ starting from $$\eta_0 = 4.6875\times 10^{-7}$$. This value is chosen such that $$2^7( 4.6875\times 10^{-7}) = 3.0\times 10^{-5}$$.
We plot $$(G_E(t) -G_0(t))/\eta^2$$ as a function of $$\eta$$. We expect a constant behavior in the region where the $$\eta^2$$ scaling is valid. The plots below show our results for the neutron at several different time slices. The parameters used in this analysis are listed in the table below.
Configuration
knhyp242448_beta7.1_kappa0.1282_023
ran on Fermilab.
| CG precision | 1e-8 | 1e-13 |
|---|---|---|
| $$\kappa$$ | 0.1282 | 0.1282 |
| n iterations ~ | 700 | 1250 |
| timing for 1 inversion ~ | 15 sec | 22 sec |
| N GPUs | 2 | 4 |